Experiment 5 - Impact of a Jet

Object

To compare the momentum in a fluid jet with the force generated when the jet strikes a flat plate and a hemispherical cup.

Apparatus

The following equioment is required for this experiment:

1. Impact of a jet Apparatus.
2. Steady water supply with a flow control valve.
3. Flow meter.
4. A flat plate and an hemispherical cup.
5. Set of calibrated weights.

The impact of a jet apparatus is depicted in Figure 5.1. The jet and plate are located inside a transparent casing.

Figure 5.1.

Theory

Applying the momentum equation is the x direction:

-F_x = p Q [ v_{x, out} - v_{x, in} ] = p Q [ v cos ß - v ]

F_x = p Q v [ 1 - cos ß ]

For the flat plate ß = 90 ° and

F_x = p Q v

For the hemispherical cup ß = 180 ° and

F_x = 2 p Q v

Figure 5.2.

The exit velocity, u can be obtained from the flow rate Q as follows:

u = q ÷ area of nozzle

The jet at the nozzle is vertically displaced from the point of contact on the plate and therefore an the exit velocity, u, can be related to the impact velocity, v.

Applying the Bernoulli equation between nozzle and plate:

( P_n ÷ gamma ) + ( u² ÷ [ 2 g ]) + Z_n = ( P_p ÷ gamma ) + ( v² ÷ [ 2 g ]) + Z_p

Since the jet is open to the atmosphere

( P_n ÷ gamma ) - ( P_p ÷ gamma ) = 0

and

Z_n - Z_p = s

Therefore

v² = u² - 2 g s

Experimental procedure

The procedure outlined in steps 1 through 7 is to be followed for this experiment.

1. Install the flat plate in the apparatus.
2. Note the no load position of the weigh tray by aligning the pointer at the top of the apparatus with the weigh pan.
3. Adjust the flow rate to just below the maximum value that can be measured by the flow meter.
4. Add the appropriate masses to the weigh tray until it returns to the no load position.
5. Record the flow rate and mass.
6. Reduce the water supply and repeat the procedure (steps 4 and 5) for each flow rate.
7. Replace the flat plate with an hemispherical cup and repeat the procedure (steps 2-6).

Report

In the report:

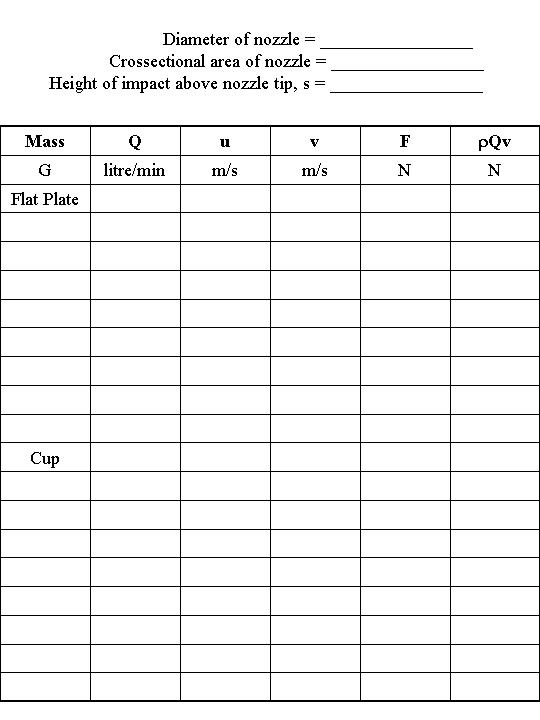
1. Determine the force on the plate for each flow rate.
2. Compute the force on the flat plate by the rate of delivery of momentum (density × Q × v) of the jet at impact.
3. Plot the force on the flat plate against the delivery of the momentum of the jet. Derive an equation for force on the flat plate by means of this graph.
4. Compare the results with the analytical solution for the for4ce on the flat plate assuming no fluid friction.
5. Repeat steps 1 to 4 for the hemispherical cup.
6. Discuss your results.

Table 5.1