ENGI 5432 Advanced Calculus
Faculty of Engineering and Applied Science2009 Winter
Problem Set 2 - Solutions
- For the vector field
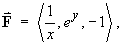
- find the equations of the lines of force and
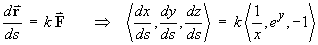
Þ k ds = x dx = e -y dy = -dz
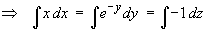
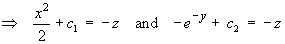
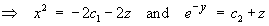
In order to avoid the problem of multiple square roots, make x the parameter
Then,
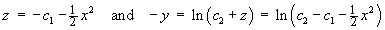
Let A = c 2 - c 1 and B = -c1 then the lines of force are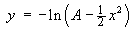 ,
,
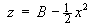 (provided x2 < 2A).
(provided x2 < 2A).Alternate forms for the answer include
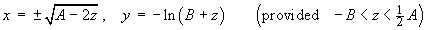
and
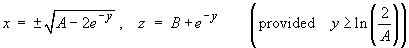
- find the equations of the particular line of force passing
through the point
(2, 0, 4).
The line of force passes through (2, 0, 4)
Þ 0 = -ln(A - 4/2) Þ A - 2 = 1 Þ A = 3
and 4 = B - 4/2 Þ B = 4 + 2 = 6
Therefore the line of force is ,
,
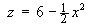 (provided x2 < 6)
(provided x2 < 6) or
with z as the parameter
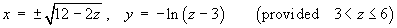
or
with y as the parameter
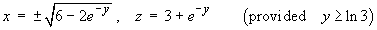
- find the equations of the lines of force and
- For the vector field
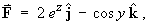
- find the equations of the lines of force and

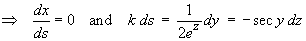

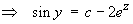
Therefore the lines of force are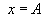 ,
,


or
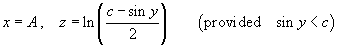
- find the equations of the particular line of force passing
through the point
(3, p, 0).
The line of force passes through (3, p, 0)
Þ 3 = A and sin p = c - 2e0
Þ 0 = c - 2 Þ c = 2
Therefore the line of force is
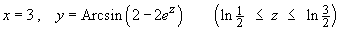
or
with y as the parameter: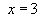 ,
,
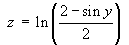 (for all real y)
(for all real y)
- find the equations of the lines of force and
Find the family of vector fields in
 whose lines of force are straight lines.
whose lines of force are straight lines.
With s = arc length and
 constant vectors, the vector parametric form of
constant vectors, the vector parametric form of
the equation of a straight line can be written as
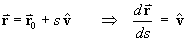
But, along a line of force,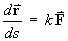
Therefore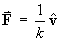 ,
which is parallel to
,
which is parallel to

Þ F must be a family of parallel vectors:
(the direction never changes, although the magnitude may vary)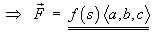 ,
,where a, b, c = scalar constants (not all zero) and
f (s) = some scalar function of s.
Any vector field of this type is a valid solution.Check [not required]:
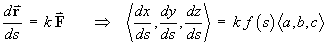
If any of a, b, c is zero, then the corresponding coordinate (x, y or z) is constant
along the line of force. For the non-zero case,
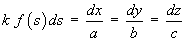 , whose solution is
, whose solution is
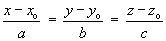 ,
(where (xo , yo ,
zo ) is any point on the line of force),
,
(where (xo , yo ,
zo ) is any point on the line of force),
which is a straight line.
When exactly one of a, b, c is zero, the line is parallel to the corresponding coordinate plane.
When two of a, b, c are zero, the line is parallel to the remaining coordinate axis.
The plane P passes through the point
(2, -1, 5) and contains the lineL : y = -3 , z = x - 2 . - Find the Cartesian equation of the plane
P.
![[diagram]](p2/q4sketch.gif)
L : y = -3 , z = x - 2
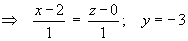
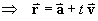 where
where
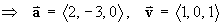
Let Q be (2, -3, 0) (which a point known to be on the line)
Let P be (2, -1, 5) and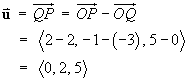
The required plane contains both of the vectors u and v.
The plane normal is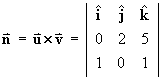
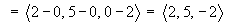
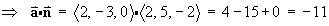
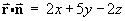
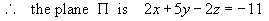
or 2x + 5y - 2z + 11 = 0
- Find the Cartesian equations of the normal line to
P that passes through the
origin.
Normal Line:
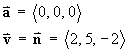 (line must pass through origin)
(line must pass through origin)
therefore the line is
- Find the Cartesian equation of the plane
P.
Find the [acute] angle q (to the nearest 0.1°) between the line
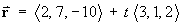
and the plane

![[diagram]](p2/q5sketch.gif)

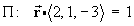
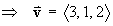
and
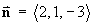

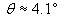
A temperature distribution for a region within 75 metres of the origin is given by
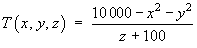
- Find the gradient of the temperature function T.
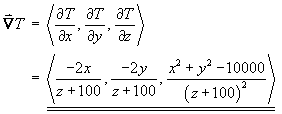
- Find the [instantaneous] rate at which the temperature is changing
at the point
(50, 50, 0) in the same direction as the vectori - j .
P is the point (50, 50, 0)
Direction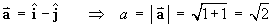
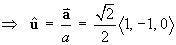

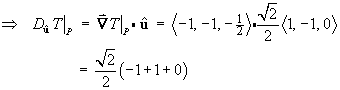
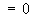
[The vector i - j is at right angles to the gradient vector (and therefore tangential to a level surface) at the point (50, 50, 0).]
- Is the field formed by the gradient vector purely radial?
[That is, does the gradient vector point directly towards or directly away from the origin at every point?]
At P (50, 50, 0),
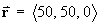 but
but
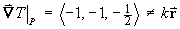 for any scalar k.
for any scalar k.
Therefore,No , the gradient vector is not purely radial.
- Find the gradient of the temperature function T.
Find the equations of the tangent plane and the normal line to the sphere
x2 + y2 + z2 = 9
at the point (-2, 1, 2).
f (x, y, z) = x2 + y2 + z2 = 9
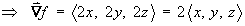
Use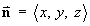
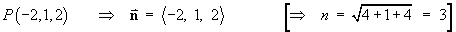
Tangent plane at P:
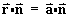
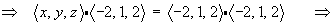

Normal line at P:

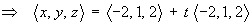
[from which is it easy to deduce that this normal line passes through the origin.]
One form of the equations of the normal line is