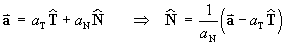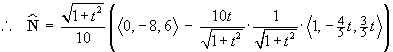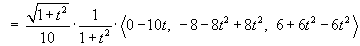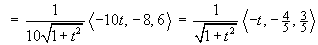ENGI 5432 Advanced Calculus
Faculty of Engineering and Applied Science2009 Winter
Problem Set 3 - Solutions
- A curve is defined in vector parametric form by
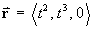
- Show that the unit tangent is well-defined everywhere along the
curve except at the origin.
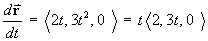
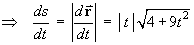
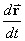 and
and
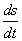 are
well-defined everywhere, but
are
well-defined everywhere, but
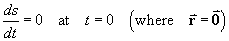

is well-defined everywhere except at the origin (0, 0, 0). - Eliminate the parameter t to find the Cartesian equations
of the curve.
x = t2 y = t 3 z = 0

Therefore the equations of the curve are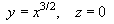
- Sketch the curve. Display on your sketch the unit tangent
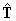 and the unit principal normal
and the unit principal normal
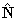 at two points
close to the origin, one in the region where
at two points
close to the origin, one in the region where
t < 0 and the other in the region wheret > 0 .
![[graph showing cusp]](p3/q1cgraph.gif)
- From your sketch, state briefly why the unit tangent is not
defined at the origin.
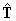 “bounces” from
“bounces” from
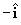 to
to
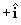 abruptly
at t = 0
abruptly
at t = 0
(and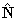 also changes
abruptly at t = 0, from
also changes
abruptly at t = 0, from
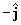 to
to
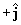 )
) - From your sketch, show that the unit binormal
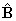 has a well
defined limit at the origin, (even though the unit tangent
and unit principal normal do not).
has a well
defined limit at the origin, (even though the unit tangent
and unit principal normal do not).
Everywhere except t = 0,
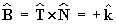
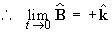
- Show that the unit tangent is well-defined everywhere along the
curve except at the origin.
For the surface whose equation in cartesian coordinates is
z2 = x2 - y2, - what type of quadric surface is this surface?
![[Maple plot of cone]](p3/cone1.gif) z2 = x2
- y2
Þ
-
x2 + y2 +
z2 = 0
z2 = x2
- y2
Þ
-
x2 + y2 +
z2 = 0
This quadric surface is of the type with a = b
with a = b
It is therefore acircular cone whose axis of symmetry is the x-axis
- find the cartesian equation of the tangent plane to the surface
at the point
(1, 1, 0).
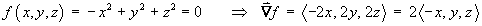
At (1,1,0)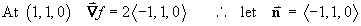
(Any non-zero multiple of the gradient vector is a normal vector.)
A point on the tangent plane is (1, 1, 0)

Þ the tangent plane is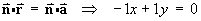 or
or
- find the cartesian equations of the normal line to the surface at
the point
(1, 1, 0).
Normal line:
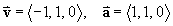
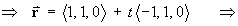
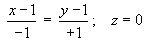
or y = 2 - x, z = 0
[Note that the line y = x, z = 0 is one of the generators of the circular cone.]
- what type of quadric surface is this surface?
For the surfaces whose cartesian equations are z = 3x2 + 2y2 and
-2x + 7y2 - z = 0 : - what types of quadric surface are these surfaces?
![[Maple plot of parabolic cylinder
intersecting elliptic paraboid]](p3/ellpar1.gif) z = 3x2 + 2y2
is of type
z = 3x2 + 2y2
is of type
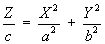 elliptic paraboloid
elliptic paraboloid
with its axis of symmetry along the z-axis.-2x + 7y2 - z = 0 Þ 7y2 = +2x + z
is of type parabolic cylinder
parabolic cylinder
Its vertex line is 2x + z = 0, y = 0 - find the angle between the surfaces at the point (1, 1, 5).

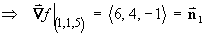
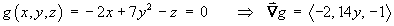

[One should check that (1, 1, 5) does indeed lie on both surfaces]
q = Angle between surfaces = Angle between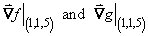
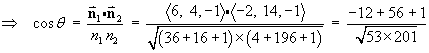
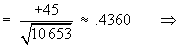
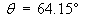
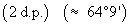
- what types of quadric surface are these surfaces?
Calculate the directional derivative of
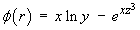 at the point
at the point
(5, 1, -2) in the direction of the vector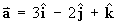 .
.
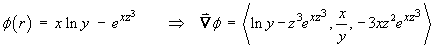
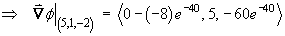
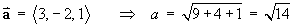
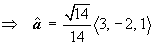

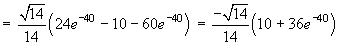
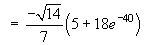
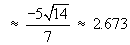
Given that the velocity of a particle at any time t is
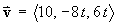 and that the particle is at the origin when
and that the particle is at the origin when
t = 0 , find the unit binormal vector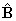 to the curve along which the particle moves and
hence find the equation of the plane in which the trajectory
of the particle lies.
to the curve along which the particle moves and
hence find the equation of the plane in which the trajectory
of the particle lies.
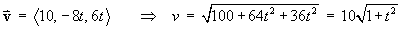
![==> T-hat = [1/( 10(1+t^2)^.5)] < 10,-8t,6t >
= [1 / (1+t^2)] < 1, -4t/5, 3t/5 >](p3/q5_sol_3.gif)
![==> dT-hat/dt = [ <0,-4/5,3/5 >(1+t^2) -
< 1, -4t/5,3t/5 > (t/(1+t^2).5) ]/1+t^2](p3/q5_sol_4.gif)
![=[ <0-t,-45/-4t^2/5+4t^2/5, 3/5+3t^2/5-3t^2/5 > ]
/ (1+t^2)^(3/2) = [ < -t, -4/5, 3/5 > ] / (1+t^2)^(3/2)](p3/q5_sol_5.gif)
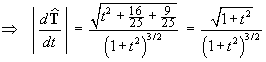
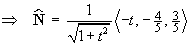
![==> B-hat = T-hat cross N-hat
= (1 / (1+t^2)) * [ i j k; 1 -4t/5 3t/5; -t -4/5 3/5]](p3/q5_sol_8.gif)
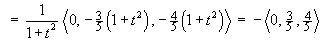
Therefore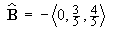 everywhere along the curve
everywhere along the curve
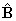 = constant
Þ
the curve lies in one plane
= constant
Þ
the curve lies in one plane
The plane normal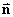 = any non-zero multiple of
= any non-zero multiple of
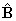 .
.
Choose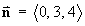
The curve passes through the origin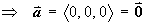
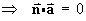
The equation of the plane is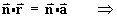
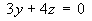
[Note: the curve is a parabola in this plane.]
Alternative Calculation of Unit Principal Normal
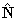 :
:Use the acceleration vector:
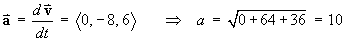
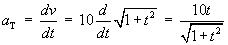
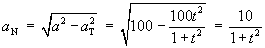
But