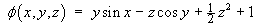ENGI 5432 Advanced Calculus
Faculty of Engineering and Applied Science2009 Winter
Term Test 2 - Solutions
For the vector field defined by
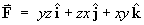 ,
,- Find the divergence of
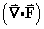
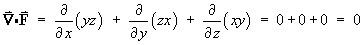

- Hence find the total flux
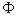 of
of
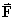 through the sphere of radius 1, centre
through the sphere of radius 1, centre
(0, 0, 0).
Using Gauss’ divergence theorem,
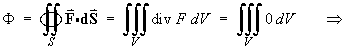
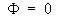
[Note that the details of the sphere are irrelevant - for this source-free vector field, the net flux through any simple closed surface will be zero!]
- Find the divergence of
In the x-y plane a vector field is defined by
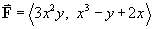 .
.- Use Green’s theorem to find the work done by
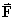 in one circuit around the unit square (vertices
(0, 0), (1, 0), (1, 1) and
(0, 1)).
in one circuit around the unit square (vertices
(0, 0), (1, 0), (1, 1) and
(0, 1)).
![[image of unit square]](t2/q209square.gif) Green’s theorem states that, in any simply connected
domain,
Green’s theorem states that, in any simply connected
domain,

where C is a simple closed path that encloses the region D.
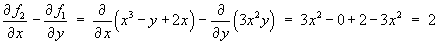

But D is a unit square, whose area is A = 1. Therefore
- Is the vector field
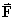 conservative? Why or why not?
conservative? Why or why not?
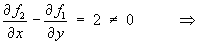
NO, F is not conservative.
- Use Green’s theorem to find the work done by
The velocity field of a fluid is
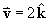 .
Find the flux Q of the fluid through
the hemisphere
.
Find the flux Q of the fluid through
the hemisphere
 in a direction outward from its centre.
in a direction outward from its centre.
[Hint: use a spherical polar coordinate grid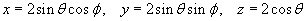 , with
, with
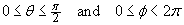
![[image of hemisphere]](t2/hemisphere.gif)

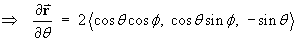
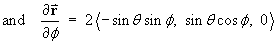

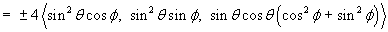
But, everywhere on the hemisphere,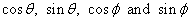 are all positive.
are all positive.
For an outward pointing normal vector (pointing away from the origin), we must select the '+' sign.
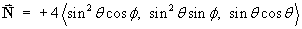
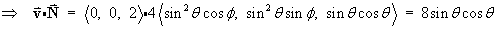
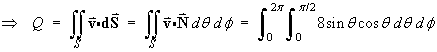

Therefore the flux is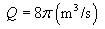
BONUS QUESTION
Find the potential function
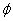 for
for
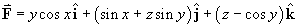 such that
such that
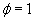 everywhere along the y-axis.
everywhere along the y-axis.
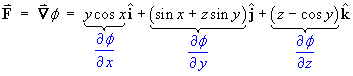
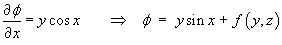
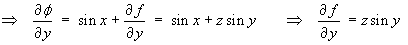
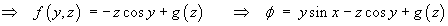

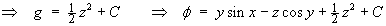
But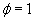 everywhere along the y-axis
everywhere along the y-axis
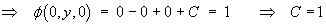
Therefore the required potential function is